RS Aggarwal Class 8 Math Fifth Chapter Playing with Numbers Exercise 5C Solution

RS Aggarwal Class 8 Math Fifth Chapter Playing with Numbers Exercise 5C Solution

EXERCISE 5C

Replace A, B, C by suitable numerals.

(1) Solution: A + 7 = 13

⇒ A = 13 – 7 = 6

So, 1 is carried over.

(1 + 5 + 8) = 14. So, B = 4 and 1 is carried over.

And C = 1.

∴ A = 6, B = 4 and C = 1.

(2) Solution: A + 6 = 13

⇒ A = 16 – 6 = 7

So, 1 is carried over.

1 + B + 9 = 17

⇒ B = 17 – 10 = 7, B = 7 and 1 is carried over.

1 + C + 6 = 11

⇒ C = 11 – 7 = 4.

∴ A = 7, B = 7 and C = 4.

(3) Solution: A + A + A = A, 1 is carried over.

When, A = 5

A + A + A = 15, (1 is carried over)

⇒ B = 1.

∴ A = 5, B = 1.

(4) Solution: 6 – A = 3

This implies that the maximum value of A can be 3.

A ≤ 3       …..(i)

The next column has the following:

A – B = 7

To reconcile this with equation (1), borrowing is involved.

We know: 12 – 5 = 7

∴ A = 2 and b = 5

(5) 5 – A = 9

This implies that 1 is borrowed.

We know, 15 – 6 = 9

⇒ A = 6

B – 5 = 8

This implies that 1 is borrowed.

13 – 5 = 8

But 1 has also been lent.

∴ B = 4

C – 2 = 2

This implies that 1 has been lent.

∴ C = 5

∴ A = 6, B = 4 and C = 5.

(6) (B × 3) = B

Then, B can either be 0 or 5.

If B is 5, then 1 will be carried.

Then, A × 3 + 1 = A will not be possible for any number.

∴ B = 0

A × 3 = A is possible for either 0 or 5.

If we take A = 0, then all number will become 0. However, this not a possible.

∴ A = 5

Then, 1 will be carried.

∴ C = 1

∴ A = 5, B = 0 and C = 1

(7) A × B = B

⇒ A = 1

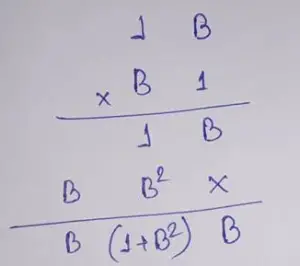
In the question:

First digit = B + 1

Thus, 1 will be carried from 1 + B² and becomes (B + 1) (B² – 9)B.

∴ C = B² – 1

Now, all b, B + 1 and B² – 9 are one digit number.

This condition is satisfied for B = 3 or B = 4.

For, B<3, B – 9 will be negative.

For, B > 3, B² – 9 will become digit number.

For B = 3, C = 3² – 9 = 9 – 9 = 0

For, B = 4, C = 4² – 9 = 16 – 9 = 7

∴ A = 1, B = 4, C = 7.

(8) Solution: (A – 4) = 3

⇒ A = 7

Also, 6 × 6 = 36

⇒ 36 – 36 = 0

⇒ B = 6

∴ A = 7

B = C = 6.

(9) Find two numbers whose product is a 1- digit number and the sum is a 2-digit number.

Solution: 1 and 9 are two numbers whose product is a single digit number.

∴ 1 × 9 = 9

Sum of the numbers is a two digit number.

∴ 1 + 9 = 10

(10) Find three whole numbers whose product and sum are equal.

Solution: The three whole numbers are 1, 2 and 3.

1 + 2 + 3 = 6 = 1 × 2 × 3

(11) complete the magic square given below, so that the sum of the numbers in each roe or in each column or along each diagonal is 15.

6	1
	5

Solution: 6 + 5 + x = 15

⇒ x = 4

Now taking the first row:

6 + 1 + x = 15

⇒ x = 15 – 7 = 8

Taking last column:

8 + x + 4 = 15

⇒ x = 15 – 12 = 3

Taking second column:

1 + 5 + x = 15

⇒ x = 15 – 6 = 9

Taking second row:

X + 5 + 3 = 15

⇒ x = 15 – 8 = 7

Taking diagonal that begins with 8:

8 + 5 + x = 15

⇒ x = 2

6	1	8
7	5	3
2	9	4

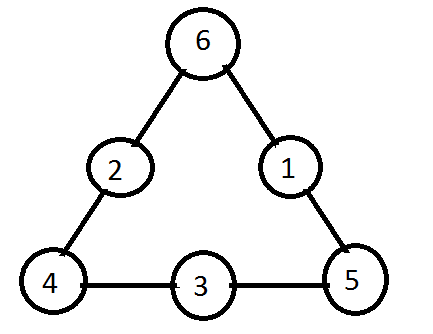
(12) Fill in the numbers from 1 to 6 without repetition, so that each side of the triangle adds up to 12.

Solution: 6 + 2 + 4 = 12

4 + 3 + 5 = 12

6 + 1 + 5 = 12

(13) Fibonacci numbers Take 10 numbers shown below:

A, b, (a + b), (a + 2b), (2a + 3b), (3a + 5b), (5a + 8b), (8a + 13b), (13a + 21b), and (21a + 34b). Sum of all these numbers = 11(5a + 8b) = 11 × 7^th number.

Solution: a = 8 and b = 13

The numbers are 8, 13, 21, 34, 55, 89, 144, 233, 377 and 610.

Sum of the numbers = 8 + 13 + 21 + 34 + 55+ 89 + 144 + 233 + 377 + 610 = 1584

11 × 7th number = 11 × 144 = 1584

(14) Complete the magic square:

	14		0
8		6	11
4			7
	2	1	12

Solution: The magic square is completed assuming that the sum of the row, columns and diagonals is 30. This is because the sum of all the number of the last column is 30.

3	14	13	0
8	5	6	11
4	9	10	7
15	2	1	12