RS Aggarwal Class 8 Math Eighth Chapter Linear Equations Exercise 8B Solution
EXERCISE 8B
(1) Two numbers are in the ratio 8 : 3. If the sum of the numbers is 143, find the numbers.
Solution: Let the two number is 8x and 3x respectively.
∴ 8x + 3x = 143
⇒ 11x = 143
⇒ x = 13
Therefore, the numbers are (8 × 13) = 104 and (3 × 13) = 39.
(2) 2/3 of numbers is 20 less than the original numbers. Find the number.
Solution: Let the original number be x.
∴ (2/3) x = x – 20
⇒ 2x = 3x – 60
⇒ 2x – 3x = – 60
⇒ – x = – 60
⇒ x = 60
Therefore, the number is 60.
(3) Four – fifths of a number is 10 more than two – thirds of the number. Find the number.
Solution: Let the number be x.
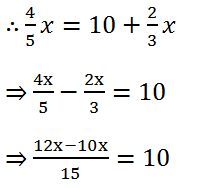
⇒ 2x = 150
⇒ x = 75
Therefore, the number is 75.
(4) Twenty – four is divided into two parts such that 7 times the first part added to 5 times the second part makes 146. Find each part.
Solution: Let the part be x.
7 times added first part = 7x
And the other part be = (24 – x)
5 times the second part = 5 (24 – x)
∴ 7x + 5(24 – x) = 146
⇒ 7x + 120 – 5x = 146
⇒ 2x = 146 – 120
⇒ x = 26/2
⇒ x = 13
Therefore, the each part is 13.
(5) Find the number whose fifth part increased by 5 is equal to its fourth part diminished by 5.
Solution: Let the number be x.
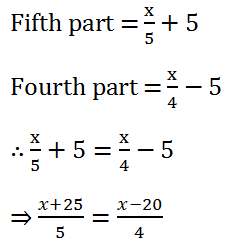
⇒ 5x – 100 = 4x + 100
⇒ 5x – 4x = 100 + 100
⇒ x = 200
Therefore, the number is 200.
(6) Three numbers are in the ratio 4 : 5 : 6. If the sum of the largest and the smallest equals the sum of the third and 55, find the numbers.
Solution: Let the numbers be 4x, 5x and 6x respectively
∴ 4x + 6x = 55 + 5x
⇒ 10x – 5x = 55
⇒ 5x = 55
⇒ x = 11
Therefore, the numbers are (4 × 11) = 44, (5 × 11) = 55 and (6 × 11) = 66.
(7) If 10 be added to four times a certain number, the result is 5 less than five times the number. Find the number.
Solution: Let the number be x.
∴ 10 + 4x = 5x – 5
⇒ 4x – 5x = – 5 – 10
⇒ – x = – 15
⇒ x = 15
Therefore, the number is 15.
(8) Two numbers are such that the ratio between them is 3 : 5. If each is increased by 10, the ratio between the new numbers so formed is 5 : 7. Find the original numbers.
Solution: Let the numbers be 3x and 5x respectively.

Therefore, the required number is 5.
First number = (3 × 5) = 15 and second number = (5 × 5) = 25.
(9) Find three consecutive odd numbers whose sum is 147.
Solution: Let the three consecutive odd number be x, (x + 2) and (x + 4).
∴ x + x + 2 +x + 4 = 147
⇒ 3x + 6 = 147
⇒ 3x = 147 – 6
⇒ 3x = 141
⇒ x = 47
Therefore, the numbers are 47, (47 + 2) = 49 and (47 + 4) = 51.
(10) Find three consecutive even numbers sum 234.
Solution: Let the three consecutive even numbers be x, (x + 2) and (x + 4).
∴ x + x + 2 + x + 4 = 234
⇒ 3x + 6 = 234
⇒ 3x = 234 – 6
⇒ 3x = 228
⇒ x = 76
Therefore, the three numbers are 76, (76 + 2) = 78 and (76 + 4) = 90.
(11) The sum of the digits of a two – digit number is 12. If the new number formed by reversing the digits is greater than the original number by 54, find the original number. Check your solution.
Solution: Let the required number be x.
Digit of 10 place = (12 – x)
∴ Original number = 10(12 – x) + x = 120 – 10x + x = 120 – 9x
New number = 10x + 12 – x = 9x + 12
∴ New number – Original number = 54
⇒ (9x + 12) – (120 – 9x) = 54
⇒ 9x + 12 – 120 + 9x = 54
⇒ 18x = 54 + 108
⇒ 18x = 162
⇒ x = 9
Therefore, the unit place is 9.
Digit in ten place = (12 – 9) = 3
Hence, the original number is 39.
Check the solution: The original number = 39
Sum of the digit = 3 + 9 = 12
New number = 93
New number – original number = (93 – 39) = 54.
(12) The digit in the ten place of a two – digit number is three times that in the units place. If the digits are reversed, the new number will be 36 less than original number. Find the original number. Check your solution.
Solution: Let the digit in the unit place be x.
Digit in the ten place = 3x.
Original number = (10 × 3x) + x = 31x
New number = 10x + 3x = 13x
∴ New number = Original number – 36
⇒ 13x = 31x – 36
⇒ 13x – 31x = – 36
⇒ – 18x = -36
⇒ x = 2
Therefore, the digit in unit place = 2.
Digit in the ten place = (3 × 2) = 6
Therefore the original number = 62.
Check the solution: New number + 36 = Original number.
⇒ 26 + 36 = 62.
Therefore the original number is 62.
(13) The denominator of a rational number is greater than its numerator by 7. If the numerator is increased by 17 and the denominator decreased by 6, the new number becomes 2. Find the original number.
Solution: Let the numerator be x.
The denominator is greater than the numerator by 7.
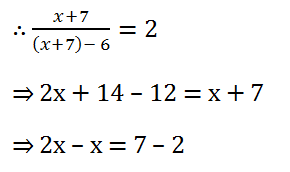
⇒ x = 5
Therefore, the numerator is 5 and the denominator is (15 + 7) = 22.
The original number is (15/22).
(14) In a fraction, twice the numerator is 2 more than the denominator. If 3 is added to the numerator and to the denominator, the new fraction is 2/3. Find the original fraction.
Solution: Let the denominator be x.
Twice of numerator = x + 2.
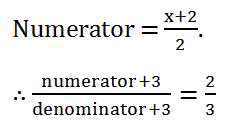
⇒ 3(numerator + 3) = 2(denominator + 3)
⇒ 3 ×
⇒
⇒ 3x + 24 = 4x+12
⇒ 3x – 4x = 12 – 24
⇒ – x = – 12
⇒ x = 12
Therefore, the denominator is 12 and the numerator = (12+2)/2=14/2=7.
∴ The original fraction = 7/12.
(15) The length of a rectangle exceeds its breadth by 7 cm. If the length is decreased by 4 cm and the breadth is increased by 3 cm, the area of the new rectangle is the same as the area of the original rectangle. Find the length and the breadth of the original rectangle.
Solution: let the breadth of the original rectangle be x cm.
The length = (x + 7) cm.
The area of the rectangle = [x × (x + 7)] sq cm.
∴ (x + 3) (x + 7 – 4) = (x) (x + 7)
⇒ x2 + 3x + 7x + 21 – 4x – 12 = x2 + 7x
⇒ x2 + 6x – x2 – 7x = – 9
⇒ – x = – 9
⇒ x = 9
Therefore, the breadth of the original number is 9 cm and the length is (9 + 7) = 16 cm.
(16) The width of a rectangle is two – thirds its length. If the perimeter is 180 metres, find the dimension of the rectangle.
Solution: Let the width of the rectangle be x cm.
The length of the rectangle be (3/2)x.
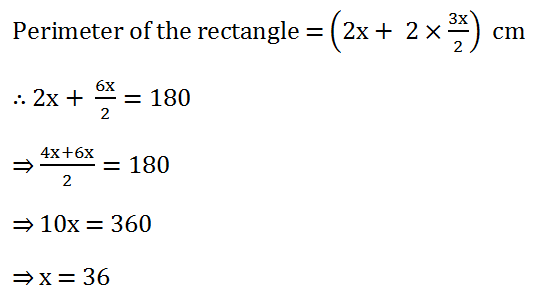
Therefore, the width of the rectangle is 36 cm.
And, the length of the rectangle =( 3/2)×36=(3×18)=54 cm.
(17) An altitude of a triangle is five – thirds the length of its corresponding base. If the altitude be increased by 4 cm and the base decreased by 2 cm, the area of the triangle remains the same. Find the base and the altitude of the triangle.
Solution: Let the length of the base be x cm.
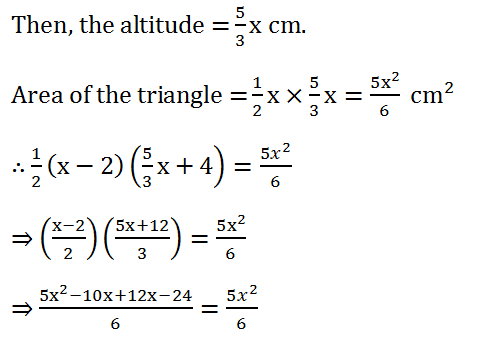
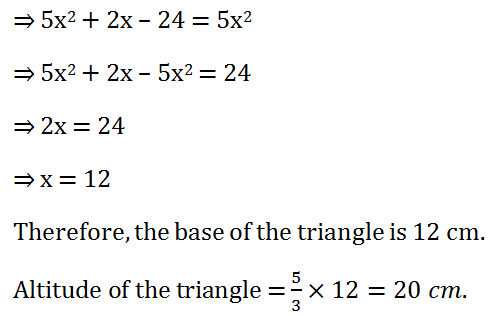
(18) Two angles of a triangle are in the ratio 4 : 5. If the sum of theses angles is equal to the third angle, find the angles of the triangle.
Solution: Let the two angle of the triangle be 4x and 5x respectively.
∴ Third angle of the triangle = 180 – (4x + 5x) = 180 – 9x.
∴ 4x + 5x = 180 – 9x
⇒ 9x = 180 – 9x
⇒ 9x + 9x = 180
⇒ 18x = 180
⇒ x = 10
Therefore, the first angle = (4 × 10) = 40o
Second angle = (5 × 10) = 50o
Third angle = (9 × 10) = 90o
(19) A steamer goes downstream from one port to another in 9 hours. It covers the same distance upstream in 10 hours. If the speed of the stream be 1 km/h, find the speed of the steamer in still water and the distance between the ports.
Solution: let the speed of the steamer in still water be x km/h.
Downstream speed = (x + 1) km/h
Upstream speed = (x – 1) km/h
Distance covered in 9 hours downstream = 9(x + 1) km
Distance covered in 10 hours upstream = 10(x – 1) km
Here given, both of these distances will be same:
9 (x + 1) = 10(x – 1)
⇒ 9x + 9 = 10x – 10
⇒ 9x – 10x = – 10 – 9
⇒ – x = – 19
⇒ x = 19
Therefore, the speed of the steamer in still water is 19 km/h.
Distance between the ports = 9(19 + 1) = 9 × 20 = 180 km
(20) The distance between two stations is 300 km. Two motorcyclists start simultaneously from these stations and move towards each other. The speed of one of them is 7 km/h more than that of the other. If the distance between them after 2 hours of their start is 34 km, find the speed of each motorcyclist. Check your solution.
Solution: let the speed of 1st motorcyclist be x km/h.
So, the speed of the 2nd motorcyclist will be (x + 7) km/h.
Distance travelled by the 1st motorcyclist in 2 hours = 2 (x + 7) km
∴ 300 – [2x + (2x + 14)] = 34
⇒ 300 – 2x – 2x – 14 = 34
⇒ 286 – 4x = 34
⇒ – 4x = 34 – 286
⇒ – 4x = – 252
⇒ x = 63
Therefore, the speed of the first motorcyclist is 63 km/h.
The speed of the 2nd motorcyclist is (63 + 7) = 70 km/h.
Check the solution:
The distance covered by the first motorcyclist in 2 hours = 63 × 2 = 126 km
The distance covered by the 2nd motorcyclist in 2 hours = 70 × 2 = 140 km.
The distance between the motorcyclist after 2 hours = 300 – (126 + 140) = 300 – 266 = 34 km
Therefore, the speeds of the motorcyclist s are 63 km/h and 70 km/h, respectively.
(21) Divide 150 into three parts such that the second number is five-sixths the first and the third number is four-fifths the second.
Solution: let the first number be x.
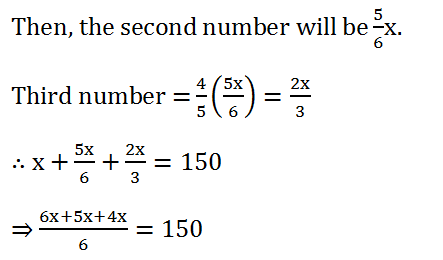
⇒ 15x = 150 × 6
⇒ 15x = 900
⇒ x = 60
Therefore, the first number is 60.
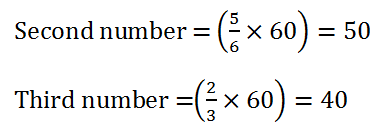
(22) Divide 4500 into two parts such that 5% of the first part is equal to 10% of the second part.
Solution: let the first part be x.
Let the second part be (4500 – x).
∴ 5% of x = 10% of (4500 – x)
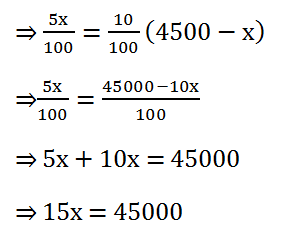
⇒ x = 3000
Therefore, the first part is 3000 and second part = (45000 – 3000) = 1500.
(23) Rakhi’s mother is four times as old as Rakhi. After 5 years, her mother will be three times as old as she will be then. Find their present ages.
Solution: Let the present age of Rakhi be x.
Then, the present age of the rakhi’s mother will be 4x.
After 5 tears, Rakhi’s are will be (x + 5).
After 5 years, her mother’s age will be (4x + 6).
∴ 4x + 5 = 3(x + 5)
⇒ 4x + 5 = 3x + 15
⇒ 4x – 3x = 15 – 5
⇒ x = 10
Present age of Rakhi = 10 years.
Present age of Rakhi’s mother = (4 × 10) = 40 years.
(24) Monu’s father is 26 years younger than Monu’s grandfather and 29 years older than Monu. The sum of the ages of all the three is 135 years. What is the ages of each one of them?
Solution: Let the age of Monu’s father be x years.
The age of Monu’s grandfather will be (x + 26) years.
Then, the age of Monu will be (x – 29) years.
∴ x + (x + 26) + (x – 29) = 135
⇒ x + x + 26 + x – 29 = 135
⇒ 3x – 3 = 135
⇒ 3x = 135 + 3
⇒ 3x = 138
⇒ x = 46
Therefore, the age of Mon’s father = 46 years.
Age of the Monu’s grandfather = (46 + 26) = 72 years.
Age of Monu = (46 – 29) = 17 years.
(25) A man is 10 times older than his grandson. He is also 54 years older than him. Find their present ages.
Solution: Let the age of the grandson be x years.
Then, his grandfather’s age will be 10x.
∴ Age of the grandson = (x + 54) years
⇒ 10x = x + 54
⇒ 10x – x = 54
⇒ 9x = 54
⇒ x = 6
Therefore, the grandson’s age is 6 years and his grandfather’s age = (10 × 6) = 60 years.
(26) The difference between the ages of two cousins is 10 years. 15 years ago, if the elder one was twice as old as the younger one, find their present ages.
Solution: let the age of the younger cousin be x years.
Then, the age of the elder cousin will be (x + 10) years.
15 years ago age of the younger cousin = (x – 15) years
15 years ago age of the elder cousin = (x + 10 – 15) years = (x – 5) years
∴ (x – 5) = 2(x – 15)
⇒ x – 5 = 2x – 30
⇒ x – 2x = – 30 + 5
⇒ – x = – 25
⇒ x = 25
Therefore, the present age of the younger cousin is 25 years.
Present age of elder cousin = (25 + 10) = 35 years.
(27) Half of herd of deer are grazing in the field and three-fourths of the remaining are playing nearby. The rest 9 are drinking water from the pond. Find the number of deer in the herd.
Solution: let the number of deer in the herd be x.
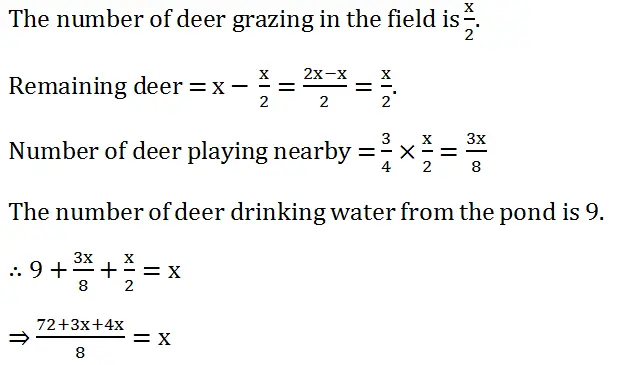
⇒ 8x = 72 + 7x
⇒ 8x – 7x = 72
⇒ x = 72.
Therefore, the total number of deer in the herd is 72.
Leave a Reply