RS Aggarwal Class 7 Math Seventeenth Chapter Constructions Exercise 17C Solution

RS Aggarwal Class 7 Math Seventeenth Chapter Constructions Exercise 17C Solution

EXERCISE 17C

OBJECTIVE QUESTIONS

Mark (√) against the correct answer in each of the following:

(1) The supplement of 45^o is

Ans: (c) 135^o

Solution: (90 + 45) = 135^o

(2) The complement of 80^o is

Ans: (b) 10^o

Solution: (90 – 80) = 10^o

(3) An angle is its own complement. The measure of the angle is         

Ans: (c) 90^o

(4) An angle is one – fifth of its supplement. The measure of the angle is

Ans: (a) 30^o

(5) An angle is 24^o more than its complement. The measure of the angle is

Ans: (b) 57^o

(6) An angle is 32^o less than its supplement. The measure of the angle is

Ans: (b) 74^o

(7) Two supplementary angles are in the ratio 3 : 2. The smaller angle measure

Ans: (c) 72^o

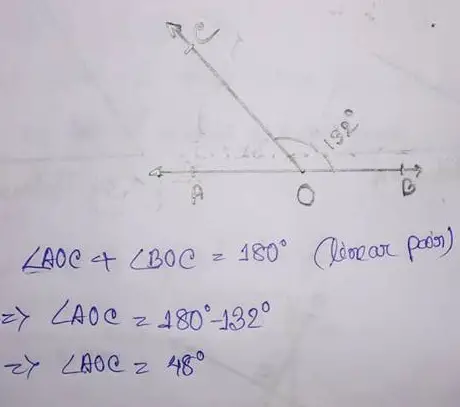
(8) In the given figure, AOB is a straight line and the ray OC stands on it.

Ans: (b) 48^o

(9) In the given figure, AOB is a straight line, ∠AOC = 68^o and ∠BOC = x^o. The value of x is

Ans: (c) 112

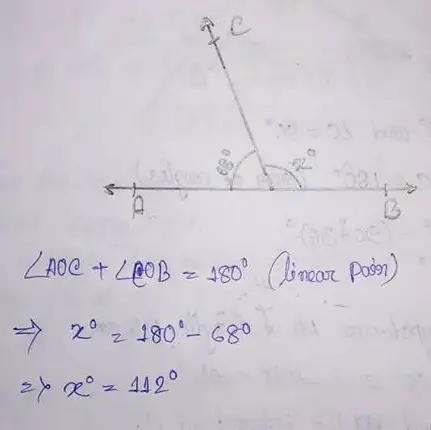
(10) In the adjoining figure, what value of x will make AOB a straight line?

Ans: (b) x = 35

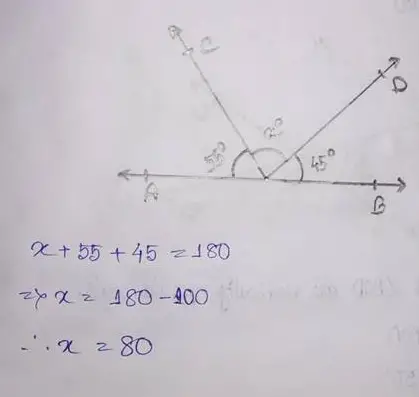
(11) In the given figure, what value of x will make AOB a straight line?

Ans: (d) x = 80

(12) In the given figure, it is given that AOB is a straight line and 4x = 5y. What is the value of x?

Ans: (a) 100

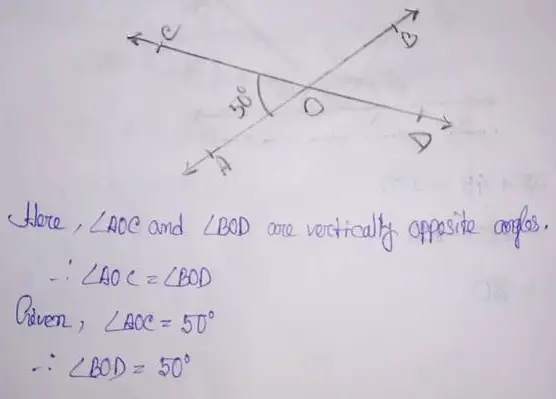
(13) In the given figure, two straight lines AB and CD intersect at a point O and ∠AOC = 50^o. Then ∠BOD =?

Ans: (b) 50^o

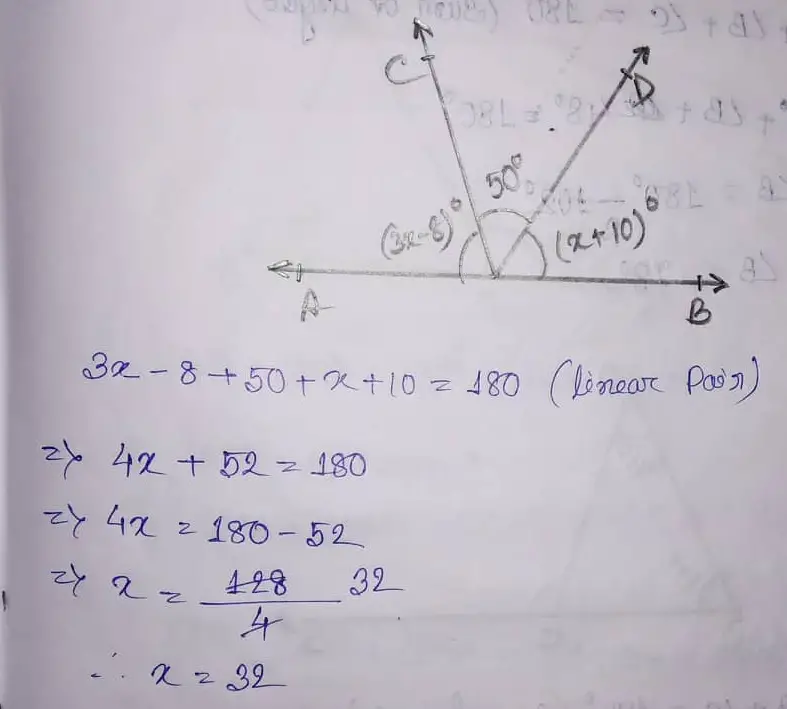
(14) In the given figure, AOB is a straight line, ∠AOC = (3x – 8)^o, ∠COD = 50^o and ∠BOD = (x+10)^o. The value of x is

Ans: (a) 32

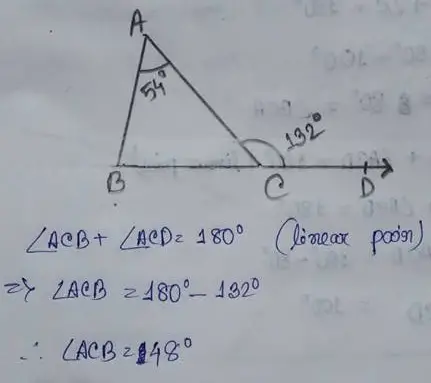
(15) In ∆ABC, side BC has been produced to D. If ∠ACD = 132^o and ∠A = 54^o. then ∠B =?

Ans: (b) 78^o

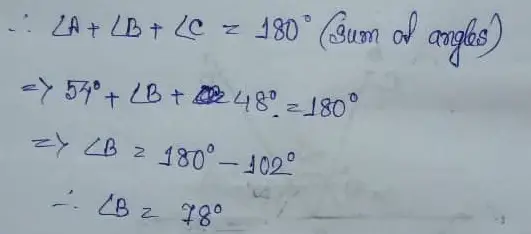
(16) In ∆ABC, side BC has been produced to D. If ∠BAC = 45^o and ∠ABC = 55^o, then ∠ACD =?

Ans: (c) 100^o

(17) In the given figure, side BC of ∆ABC is produced to D such that ∠ABC = 70^o and ∠ACD = 120^o. Then, ∠BAC =?

Ans: (b) 50^o

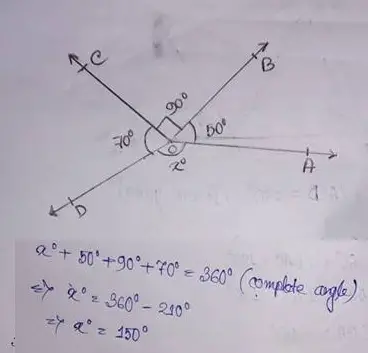
(18) In the given figure, rays OA, OB, OC and OD are such that ∠AOB = 50^o, ∠BOC = 90^o, ∠COD = 70^o and ∠AOD = x^o.

Ans: (c) 150^o 

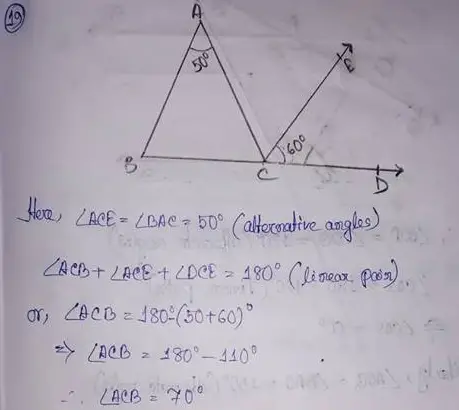
(19) In the given figure, ∠A = 50^o, CE ∥ BA and ECD = 60^o. Then, ∠ACB =?

Ans: (c) 70^o

(20) In ∆ABC, if ∠A = 65^o and ∠C = 85^o, then ∠B =?

Ans: (b) 30^o

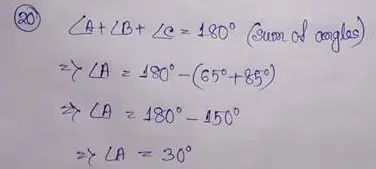
(21) The sum of all angles of a triangle is

Ans: (d) 180^o

(22) The sum of all angles of a quadrilateral is

Ans: (c) 360^o

(23) In the given figure, AB ∥ CD, ∠OAB = 150^o and ∠OCD = 120^o. Then, ∠AOC =?

Ans: (b) 90^o

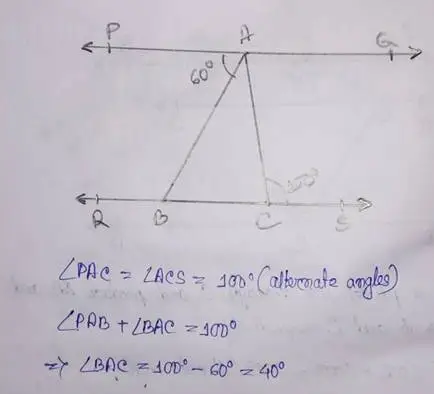
(24)In the given figure, PQ ∥ RS, ∠PAB = 60^o and ∠ACS = 100^o. Then, ∠BAC =?

Ans: (a) 40^o

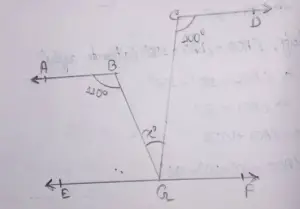
(25) In the given figure, AB ∥ CD ∥ EF, ∠ABG = 110^o, ∠GCD = 100^o and ∠BGC = x^o. Then, x =?

Ans: (c) 30

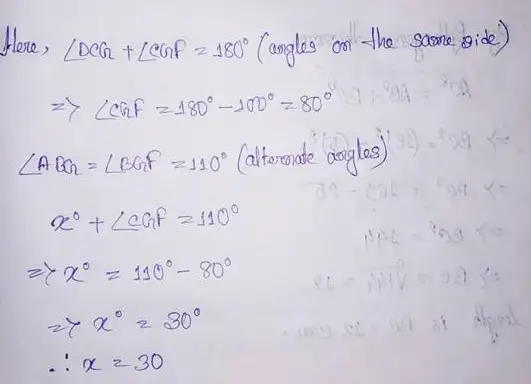
(26) The sum of any two sides of a triangle is always

Ans: (d) Greater than the 3rd side.

(27) The diagonals of a rhombus

Ans: (d) Always bisect each other at right angles.

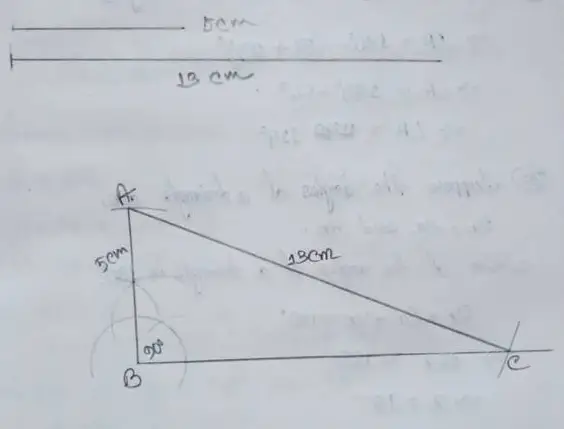
(28) In ∆ABC, ∠B = 90^o, AB = 5 cm and AC = 13 cm. Then, BC =?

Ans: (c) 12 cm

(29) In a ∆ABC it is given that ∠B = 37^o and ∠C = 29^o. Then, ∠A =?

Ans: (c) 114^o

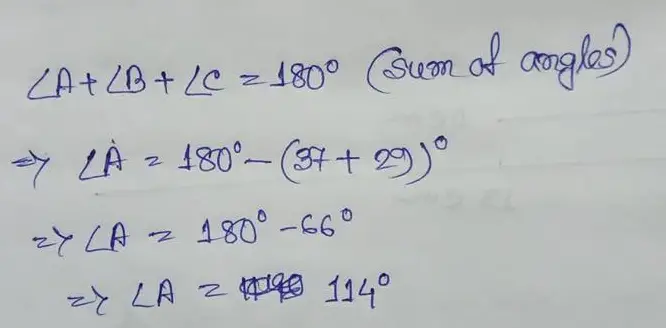
(30) The angles of a triangle are in the ratio 2 : 3 : 7. The measure of the largest angle is

Ans: (c) 105^o

(31) In a ∆ABC, if 2∠A = 3∠B = 6∠C, then ∠B =?

Ans: (c) 60^o

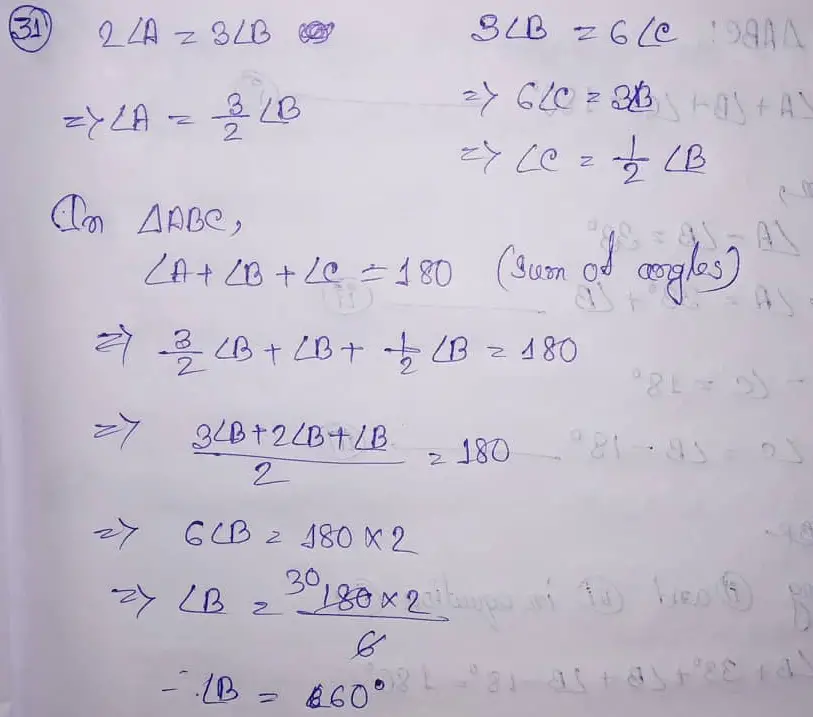
(32) In a ∆ABC, if ∠A + ∠B = 65^o and ∠B + ∠C = 140^o. Then, ∠B =?

Ans: (a) 25^o

(33) In a ∆ABC, ∠A – ∠B = 33^o and ∠B – ∠C = 18^o. Then, ∠B =?

Ans: (b) 55^o

(34) The angles of a triangle are (3x)^o, (2x – 7)^o and (4x – 11)^o. Then, x =?

Ans: (c) 22

(35) ∆ABC is right – angled at A. If AB = 24 cm and AC = 7 cm, then BC =?

Ans: (c) 25 cm

(36) A ladder is placed in such a way that its foot is 15 m away from the wall and its top reaches a window 20 m above the ground. The length of the ladder is

Ans: (b) 25 m

(37) Two poles of heights 6 m and 11 m stand vertically on a plane ground. If the distance between their feet is 12 m, what is the distance between their tops?

Ans: (a) 13 m

(38) ∆ABC is an isosceles triangle with ∠C = 90^o and AC = 5 cm. Then, AB =?

Ans: (d) 5√2 cm