S. K. Gupta Anubhuti Gangal Class 5 Math Fourth Chapter Factors And Multiples Exercise 13
EXERCISE 13
(1) Write all the factors of each of the following numbers and then tell whether it is a prime number or a composite number.
(a) 11 = 1, 11 (Prime)
(b) 32 = 1, 2, 4, 8, 16, 32 (Composite)
(c) 40 = 1, 2, 4, 5, 8, 10, 20, 40 (Composite)
(d) 19 = 1, 19 (Prime)
(e) 18 = 1, 2, 3, 6, 9, 18 (Composite)
(f) 41 = 1, 41 (Prime)
(2) Complete the factor tree. Then write the prime factorization.
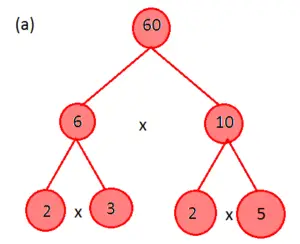
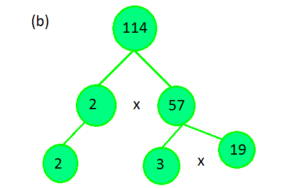
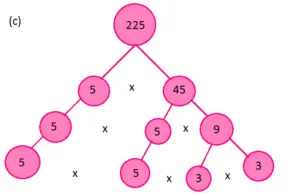
(3) Find the prime factorization of each of the following numbers.
(a) 216 = 2 × 2 × 2 × 3 × 3 × 3
(b) 150 = 2 × 3 × 5 × 5
(c) 84 = 2 × 2 × 3 ×7
(d) 1080 = 2 × 2 × 2 × 3 × 3 × 3 × 5
(4) List the common factors of each of the following sets of numbers. Hence find their HCF.
(a) 15, 35
Solution: Factors of 15 = 1, 3, 5
Factors of 35 = 1, 5, 7
The common factors are 1, 5
Out of these the common factor, 5 is largest.
∴ Therefore the HCF of 15 and 35 is 5.
(b) 16, 27
Solution: Factors of 16 = 1, 2, 4, 8
Factors of 27 = 1, 3, 9
The common factors are 1
Out of these the common factor, 1 is largest.
∴ Therefore the HCF of 16 and 27 is 1.
(c) 30, 42
Solution: Factors of 30 = 1, 2, 3, 5, 6, 10, 15
Factors of 42 = 1, 2, 3, 6, 8, 14, 21
The common factors are 1, 2, 3, 6
Out of these the common factor, 6 is largest.
∴ Therefore the HCF of 30 and 42 is 6.
(d) 18, 32, 48
Solution: Factors of 18 = 1, 2, 3, 6, 9
Factors of 32 = 1, 2, 4, 8, 16
Factors of 48 = 1, 2, 3, 4, 6, 8, 12, 16, 24
The common factors are 1, 2
Out of these the common factor, 2 is largest.
∴ Therefore the HCF of 18, 32 and 48 is 2.
(5) Find the HCF of the following by prime factorization method.
(a) 36, 60
Solution: All the factors of 36 are 2 × 2 × 3 × 3
All the factors of 60 are 2 × 2 × 3 × 5
Common factors of 36 and 60 are 2 × 2 × 3 = 12
Thus, the highest common factor of 36 and 60 is 12
Hence, HCF of 36 and 60 = 12
(b) 18, 24, 42
Solution: All the factors of 18 are 2 × 3 × 3
All the factors of 24 are 2 × 2 × 2 × 3
All the factors are of 42 are 2 × 3 × 7
Common factors of 18, 24 and 42 are 2 × 3 = 6
Thus, the highest common factor of 18, 24 and 42 is 6.
(c) 36, 48, 60
Solution: All the factors of 36 are 2 × 2 × 3 × 3
All the factors of 48 are 2 × 2 × 2 ×2 × 3
All the factors are of 60 are 2 × 2 × 3 × 5
Common factors of 36, 48 and 60 are 2 × 2 × 3 = 12.
Thus, the highest common factor of 36, 48 and 60 is 12.
(d) 28, 42, 56
Solution: All the factors of 28 are 2 × 2 × 7
All the factors of 42 are 2 × 3 × 7
All the factors are of 56 are 2 × 2 × 2 × 7
Common factors of 28, 42 and 56 are 2 × 7 = 14.
Thus, the highest common factor of 28, 42 and 56 is 14.
(6) Find the HCF of the following by long division method.
(a) 128 and 192
Solution:
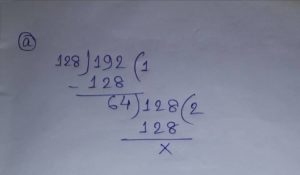
Hence, HCF of 128 and 192 is
(b) 145 and 325
Solution:
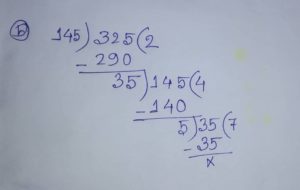
Hence, HCF of 145 and 325 is
(c) 495 and 945
Solution:
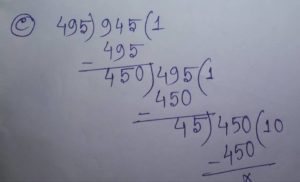
Hence, HCF of 495 and 945 is
(7) Find the LCM of the following by prime factorization method.
(a) 24 and 32
Solution: 24 = 2 × 2 × 2 × 3
32 = 2 × 2 × 2 × 2 × 2
LCM = 2 × 2 × 2 × 2 × 2 × 3 = 96
(b) 56 and 32
Solution: 56 = 2 × 2 × 2 × 7
32 = 2 × 2 × 2 × 2 × 2
LCM = 2 × 2 × 2 × 2 × 2 × 7 = 224.
(c) 25 and 40
Solution: 25 = 5 × 5
40 = 2 × 2 × 2 × 5
LCM = 2 × 2 × 2 × 5 × 5 = 200.
(8) Find the LCM of the following by division method.
(a) 4, 12 and 20
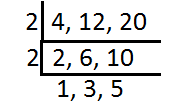
∴ LCM = 2 × 2 × 1 × 3 × 5 = 60.
(b) 16, 28, 44

∴ LCM = 2 × 2 × 4 × 7 ×11 = 1232.
(c) 39, 45, 54

∴ LCM = 3 × 3 × 13 × 5 × 6 = 3510.
(d) 25, 40, 80, 120

∴ LCM = 2 × 2 × 2 × 5 × 5 × 1 × 2 × 3 = 1200.
Leave a Reply